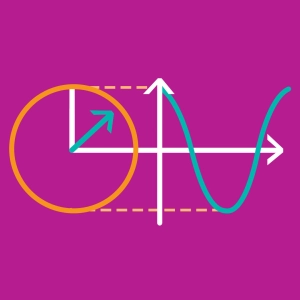
Consider a point situated on a circle with radius A. The circle has a center that coincides with the origin in the cartesian plane. Instead og using the rectangular coordinates to label this point, the x– and y- coordinates at a point on the unit circle given by an angle x (in radians) are defined by the functions x=cosx and y=sinx.

The sine function represents the shift on the y axis of the point by the function of its angle x in radians. The sinus function is defined by the equation f(x) = A sin(x).

The cosine function represents the shift on the x axis of the point by the function of its angle x. The cosine function is defined by the equation f(x) = A cos(x).

These two functions present some common characteristics:

• The values of the functions oscillate between A and -A. The parameter A corresponds to the amplitude of the function (the half-way point between the maximum and minimum values).
• The cycle repeats infinitely: the functions are periodic by period P: f (x+P) = f(x). The space between two cycles is called the period, it is also the distance between two maximums (or minimums). For the basic function, the period equals 2π radians (one complete turn of the unit circle): f(x+ 2π) = f(x).